Zalpha/2 (Zα/2)

The Zalpha/2 (Z_α/2) refers to the critical table value we obtain when conducting a two-tailed Z test at the α% level of significance. The Z_α/2 value is important because it is used to decide whether we should accept the null hypothesis or reject the null hypothesis.

Use of Z_α/2:

If the absolute value of the calculated Z statistic value exceeds Z_α/2 (Zalpha/2 or Za/2) in the two-tailed test then we reject the null hypothesis. If the absolute value of the calculated Z statistic value does not exceed Z_α/2 (Zalpha/2 or Za/2) then we accept the null hypothesis.

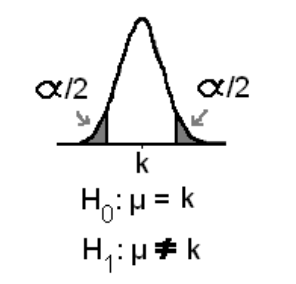
This is because Z_α/2 marks out the two critical regions at α% level of significance in the two-tailed test as shown in the below image.

Example of how to find Zalpha/2 (Z_α/2):

1. Given the level of significance α, calculate α/2.
2. Find the Z value corresponding to a probability of α/2 in the Z table.
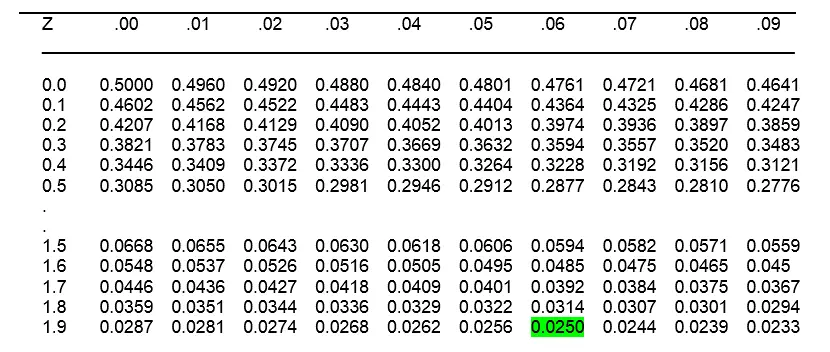
3. For example if α=5% we find that for 0.05/2=0.025 in the below table we get that Z_α/2=1.96.