The pooled standard deviation is a quantity that is used to measure the combined standard deviations for two or more samples.
- The pooled standard deviation is calculated whenever we perform two sample T tests to check for equality of means.
- Also, the pooled sample variance is used as an unbiased estimator for the combined population variance for two or more populations.
How to calculate Pooled Standard Deviation?
Suppose you have ‘k’ number of samples with each having a standard deviation of Si. The formula for pooled standard deviation is given as,
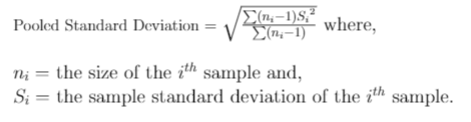
The denominator in the above formula represents the combined degrees of freedom of the test statistic.
Example:
Suppose we are given the following data about four samples. Calculate the pooled standard deviation.
| Sample size(ni) | Sample variance(Si2) |
| 4 | 12 |
| 3 | 14 |
| 6 | 16 |
| 8 | 11 |
Solution: We calculate the quantities required below,
| Sample size(ni) | Sample variance(Si2) | (ni -1) | (ni -1)* Si2 |
| 4 | 12 | 3 | 36 |
| 3 | 14 | 2 | 28 |
| 6 | 16 | 5 | 80 |
| 8 | 11 | 7 | 77 |
| ∑(ni -1)= 17 | ∑(ni -1)* Si2 = 221 |
Substituting all this in the above formula we get,
Pooled Standard Deviation = √(221/17) = √13 = 3.61